8 Origin and Heritage

One way to explain socio-cultural structures and circumstances argues from their function: if the scribe school expended much effort to teach advanced mathematics and even more on teaching Sumerian, and if it continued to do so for centuries, then these activities must have had important functions—if not as direct visible consequences then indirectly. We have just seen an explanation of that kind.

Another way to explain them—no alternative but rather the other side of the coin—is based on historical origin. Who had the idea, and when? Or, if no instantaneous invention is in focus, how did the phenomenon develop, starting from which earlier structures and conditions? In our particular case: if the invention was not made in the scribe school, where did the inspiration come from, and how did the activity perhaps change character because of the transplantation into a new environment where it came to fulfill new functions?

Over the last 40 years, our knowledge about Mesopotamian third-millennium mathematics has advanced much, in particular concerning the determination of rectangular or quasi-rectangular areas. We may now confidently assert that the reason that we have found no third-millennium texts containing algebra problems is that there were none.

This contradicts the traditional belief that everything in Mesopotamia must date from times immemorial. Certainly, we are in the “Orient” where everything, as one knows, is without age and without development (and in particular without progress)—in the “West” at least a conviction “without age and without development.”

The Origin: Surveyors’ Riddles

On the contrary, the algebra of the Old Babylonian scribe school is no continuation of century- (or millennium-)old school traditions—nothing similar had existed during the third millennium. It is one expression among others of the new scribal culture of the epoch. In principle, the algebra might have been invented within the school environment—the work on bilingual texts and the study of Sumerian grammar from an Akkadian point of view certainly were. Such an origin would fit the fact that the central vocabulary for surveying and part of that used in practical calculation is in Sumerian or at least written with Sumerian logograms (“length,” “width,” igi, “be equal by”), while the terms that characterize the algebraic genres as well as that which serves to express problems is in Akkadian.

However, an invention within the scribe school agrees very badly with other sources. In particular it is in conflict with the way problems and techniques belonging to the same family turn up in Greek and medieval sources. A precise analysis of all parallel material reveals a very different story—the material is much too vast to allow a complete presentation of the argument here, but part of it is woven into the following discussion.

The surveyors of central Iraq (perhaps a wider region, but that remains a hypothesis in as far as this early epoch is concerned) had a tradition of geometrical riddles . Such professional riddles are familiar from other pre-modern environments of mathematical practitioners (specialists of commercial computation, accounting, master builders, and of course surveying) whose formation was based on apprenticeship and not taken care of by a more or less learned school. As an example we may cite the problem of the “hundred fowls” which one finds in numerous Chinese, Indian, Arabic and European problem collections from the Middle Ages:

Somebody goes to the market and buys 100 fowls for 100 dinars. A goose costs him 3 dinars, a hen 2 dinars, and of sparrows he gets 3 for each dinar. Tell me, if you are an expert calculator, what he bought!¹

There are many solutions. 5 geese, 32 hens, and 63 sparrows; 10 geese, 24 hens, and 66 sparrows; etc. However, when answering a riddle, even a mathematical riddle, one needs not give an exhaustive solution, nor give a proof (except the numerical proof that the answer fulfills the conditions)² Who is able to give one good answer shows himself to be a competent calculator “to the stupefaction of the ignorant” (as says a manual of practical arithmetic from 1540).

Often the solution of a similar riddle asks for the application of a particular trick. Here, for instance, one may notice that one must buy 3 sparrows each time one buys a goose—that gives 4 fowls for 4 dinars—and 3 sparrows for each two hens—5 fowls for 5 dinars.

Such “recreational problems” (as they came to be called after having been adopted into a mathematical culture rooted in school, where their role was to procure mathematical fun) had a double function in the milieu where they originated. On one hand, they served training—even in today’s school, a lion that eats three math teachers an hour may be a welcome variation on kids receiving 3 sweets a day. On the other, and in particular (since the central tricks rarely served in practical computation), they allowed the members of the profession to feel like “truly expert calculators”—a parallel to what was said above on the role of Sumerian and “too advanced” mathematics for the Old Babylonian scribes.

At some moment between 2200 and 1800 bce , the Akkadian surveyors invented the trick that was later called “the Akkadian method,” that is, the quadratic completion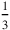; around 1800, a small number of geometrical riddles about squares, rectangles and circles circulated whose solution was based on this trick. A shared characteristic of these riddles was to consider solely elements that are directly present in the figures—for instance the side or all four sides of a square, never “3 times the area” or “ of the area.” We may say that the problems are defined without coefficients, of, alternatively, with “natural” coefficients.

If 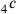stands for “the 4 sides” and 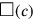for the area of a square, 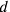for the diagonal and 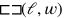for the area of a rectangle, the list of riddles seems to have encompassed the following problems:

Beyond that, there were problems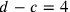 about two squares (sum of or difference between the sides given together with the sum of or difference between the areas); a problem in which the sum of the perimeter, the diameter and the area of a circle is given, and possibly the problem concerning a square, with the pseudo-solution 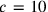, 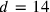; two problems about a rectangle, already known before 2200 bce, have as their data, one the area and the width, the other the area and the length. That seems to be all.³

These riddles appear to have been adopted into the Old Babylonian scribe school, where they became the starting point for the development of the algebra as a genuine discipline . Yet the school did not take over the riddle tradition as it was. A riddle, in order to provoke interest, must speak of conspicuous entities (the side, all four sides, etc.); a school institution, on the other hand, tends to engage in systematic variation of coefficients—in particular a school which, like that of the Mesopotamian scribes since the invention of writing in the fourth millennium, had always relied on very systematic variation.⁴ In a riddle it is also normal to begin with what is most naturally there (for instance the four sides of a square) and to come afterwards to derived entities (here the area). In school, on the contrary, it seems natural to privilege the procedure, and therefore to speak first of that surface which eventually is to be provided with a “projection” or a “base.”

Such considerations explain why a problem collection about squares like BM 13901 moves from a single to two and then three squares, and why all problems except the archaizing #23, “the four sides and the area,” invariably speak of areas before mentioning the sides. But the transformation does not stop there. Firstly, the introduction of coefficients asked for the introduction of a new technique, the change of scale in one direction (and then different changes in the two directions, as in TMS IX #3); the bold variation consisting in the addition of a volume and an area gave rise to a more radical innovation: the use of factorization. The invention of these new techniques made possible the solution of even more complicated problems.

On the other hand, as a consequence of the drill of systematic variation, the solution of the fundamental problems became a banality on which professional self-esteem could not be built: thereby work on complicated problems became not only a possibility but also a cultural necessity.

One may assume that the orientation of the scribal profession toward a wide range of practices invited the invention of problems outside abstract surveying geometry where the algebraic methods could be deployed—and therefore, even though “research” was no aim of the scribal school, to explore the possibilities of representation. It is thus, according to this reconstruction, the transfer to the school that gave to the cut-and-paste technique the possibility of becoming the heart of a true algebra.

Other changes were less momentous though still conspicuous. In the riddles, 10 was the preferred value for the side of the square, remaining so until the sixteenth century ce; the favorite value in school was 30′, and when an archaizing problem retained 10 it was interpreted as 10′.⁵ Finally, as explained above (page 34), the hypothetical “somebody” asking a question was replaced by a professorial “I.”

BM 13901 #23 (page 75), retaining “the four widths and the surface” (in that order) and the side 10 while changing its order of magnitude, is thus a characteristic fossil pointing to the riddle tradition. Even its language is archaizing, suggesting the ways of surveyors not educated in the scribe school. Taking into account its position toward the end of the text (#23 of 24 problems, #24 being the most intricate of all), we may see it as something like “last problem before Christmas.”

It appears that the first development of the algebraic discipline took place in the Eshnunna region, north of Babylon, during the early decades of the eighteenth century;⁶ from this area and period we have a number of mathematical texts that for once have been regularly excavated and which can therefore be dated. By then, Eshnunna was a cultural centre of the whole north-central part of Iraq; Eshnunna also produced the first law-code outside the Sumerian south. The text Db₂–146 (below, page 126) comes from a site belonging to the Eshnunna kingdom.

In c. 1761 Eshnunna was conquered by Hammurabi and destroyed. We know that Hammurabi borrowed the idea of a law-code, and can assume that he brought enslaved scholars back. Whether he also brought scholars engaged in the production or teaching of mathematics is nothing but a guess (the second-millennium strata of Babylon are deeply buried below the remains of the first-millennium world city), but in any case the former Sumerian south took up the new mathematical discipline around 1750—AO 8862 (above, page 60), with its still unsettled terminology and format, seems to represent an early specimen from this phase.

Problems from various sites in the Eshnunna region deal with many of the topics also known from later—the early rectangle variant of the “broken-reed” problem mentioned on page 70 is from one of them. Strikingly, however, there is not a single example of representation. AO 8862, on the other hand, already contains an example, in which a number of workers, their working days and the bricks they have produced are “heaped.” It does not indicate the procedure, but clearly the three magnitudes have to be represented by the sides of a rectangle and its area multiplied by a coefficient. A large part of the Eshnunna texts start “If somebody asks you thus [...],” found neither in AO 8862 nor in any later text (except as a rudiment in the archaizing BM 13901 #23).

Not much later, we have a number of texts which (to judge from their orthography) were written in the south. Several text groups obey very well-defined canons for format and terminology (not the same in all groups), demonstrating a conscious striving for regularity (the VAT- and Str-texts all belong here). However, around 1720 the whole south seceded, after which scribal culture there was reduced to a minimum; mathematics seems not to have survived. From the late seventeenth century, we have a fair number of texts from Sippar, somewhat to the north of Babylon (BM 85200+VAT 6599 is one of them), and another batch from Susa in western Iran (the TMS-texts), which according to their terminology descend from the northern type first developed in Eshnunna. And then, nothing more … .

The Heritage

Indeed, in 1595 a Hittite raid put an end to the already weak Old Babylonian state and social system. After the raid, power was grasped by the Kassites, a tribal group that had been present in Babylonia as migrant workers and marauders since Hammurabi’s times. This caused an abrupt end to the Old Babylonian epoch and its particular culture.

The scribe school disappeared. For centuries, the use of writing was strongly reduced, and even afterwards scholar-scribes were taught as apprentices within “scribal families” (apparently bloodline families, not apprenticeship formalized as adoption).

Even sophisticated mathematics disappeared. The social need for practical calculation, though reduced, did not vanish; but the professional pride of scholar-scribes now built on the appurtenance to a venerated tradition. The scribe now understood himself as somebody who knew to write, even literature , and not as a calculator; much of the socially necessary calculation may already now have rested upon specialists whose scanty literary training did not qualify them as “scribes” (in the first millennium, such a split is fairly certain).

The 1200 years that follow the collapse of the Old Babylonian cultural complex have not left a single algebra text. In itself that does not say much, since only a very small number of mathematical texts even in the vaguest sense have survived (a few accounting texts, traces of surveying, some tables of reciprocals and squares). But when a minimum of mathematical texts proper written by scholar-scribes emerges again after 400 bce, the terminology allows us to distinguish that which had been transmitted within their own environment from that which was borrowed once again from a “lay” environment. To the latter category belongs a small handful of problems about squares and rectangles. They contain no representation, no variation of coefficients, nothing sophisticated like the “broken reed” or the oil trade, only problems close to the original riddles; it would hardly be justified to speak of them as representatives of an “algebra.”

These late texts obviously do not inform us, neither directly nor indirectly, about the environment where the riddles had been transmitted, even though a continuation of the surveyors’ tradition is the most verisimilar hypothesis. Sources from classical antiquity as well as the Islamic Middle Ages at least make it clear that the tradition that had once inspired Old Babylonian algebra had survived despite the disappearance of its high-level offspring.

The best evidence is offered by an Arabic manual of practical geometry, written perhaps around 800 ce (perhaps later but with a terminology and in a tradition that points to this date), and known from a Latin twelfth-century translation.⁷ It contains all the problems ascribed above to the riddle tradition except those about two squares and the circle problem—in particular the problem about “the four sides and the area,” in the same order as BM 13901 #23, and still with solution 10 (not 10′). It also conserves the complex alternation between grammatical persons, the hypothetical “somebody” who asks the question in many of the earliest school texts, the exhortation to keep something in memory, and even the occasional justification of a step in the procedure by means of the quotation of words from the statement as something which “he” has said. Problems of the same kind turn up time and again in the following centuries—“the four sides and the area” (apparently for the last time) in Luca Pacioli’s Summa de Arithmetica from 1494, “the side and the area” of a square in Pedro Nuñez’s Libro de algebra en arithmetica y geometria from 1567 (in both cases in traditional riddle order, and in the Summa with solution 10).

In Greek mathematics, “algebraic” second-degree problems are rare but not totally absent. One is of particular interest: in one of the components of the text collection known collectively as Geometrica (attributed traditionally but mistakenly to Heron), “the four sides and the area” turns up again, though with the variation that “the four sides” have become “the perimeter.” Here, the geometric description is so precise that we can even decide the orientation of the diagram—the rectangle representing the four sides is joined below, see Figure 8.1. The text speaks explicitly of the rectangle that represents 4 as “four feet.”

Since the discovery of Babylonian algebra, it has often been claimed that one component of Greek theoretical geometry (namely, Euclid’s Elements II.1–10) should be a translation of the results of Babylonian algebra into geometric language. This idea is not unproblematic; Euclid, for example, does not solve problems but proves constructions and theorems. The geometric interpretation of the Old Babylonian technique, on the other hand, would seem to speak in favor of the hypothesis.

However, if we align the ten theorems Elements II.1–10 with the list of original riddles we make an unexpected discovery: all ten theorems can be connected directly to the list—they are indeed demonstrations that the naive methods of the riddle tradition can be justified according to the best theoretical standards of Euclid’s days. In contrast, there is nothing in Euclid that can be connected to the innovations of the Old Babylonian school. Its algebra turns out to have been a blind alley—not in spite of its high level but rather because of this level, which allowed it to survive only in the very particular Old Babylonian school environment.

The extraordinary importance of the Elements in the history of mathematics is beyond doubt. None the less, the most important influence of the surveyors’ tradition in modern mathematics is due to its interaction with medieval Arabic algebra.

Even Arabic algebra seems to have originally drawn on a riddle tradition. As mentioned above (page 92), its fundamental equations deal with an amount of money (a “possession”) and its square root. They were solved according to rules without proof, like this one for the case “a possession and ten of its roots are made equal to 39 dinars”:

you halve the roots, which in this question are 5. You then multiply them with themselves, from which arises 25; add them to 39, and they will be 64. You should take the root of this, which is 8. Next remove from it the half of the roots, which is 5. Then 3 remains, which is the root of the possession. And the possession is 9.

Already the first author of a treatise on algebra which we know (which is probably the first treatise about the topic⁸)—al-Khwārizmī, from the earlier ninth century ce—was not satisfied with rules that are not based on reasoning or proof. He therefore adopted the geometric proofs of the surveyors’ tradition corresponding to Figures 3.1, 3.3, 4.1 and, first of all, the characteristic configuration of Figure 4.12. Later, mathematicians like Fibonacci, Luca Pacioli and Cardano saw these proofs as the very essence of algebra, not knowing about the polynomial algebra created by al-Karajī, as-Samaw’al and their successors (another magnificent blind alley). In this way the old surveyors’ tradition conquered the discipline from within; the word census, the Latin translation of “possession,” came to be understood as another word for “square.” All of this happened in interaction with Elements II—equally in debt to the surveyors’ tradition, as we have just seen.

Thus, even though the algebra of the cuneiform tablets was a blind alley—glorious but blind all the same—the principles that it had borrowed from practitioners without erudition was not. Without this inspiration it is difficult to see how modern mathematics could have arisen. As has been said about God: “If he did not exist, one would have had to invent him.”

Footnotes