7 The Background

What we now know about Old Babylonian algebra—its flexibility, its operational power in the solution of sophisticated though practically irrelevant problems, the competence of those who practised it—leaves unanswered the enigma of its existence. Since this enigma is now almost 4000 years old, we may hope to learn something about our own epoch through a reflection on the situation in king Hammurabi’s century.

The Scribe School

Old Babylonian mathematics was not the high-status diversion of wealthy and highly intelligent amateurs, as Greek mathematicians were or aspired to be. According to the format of its texts it was taught in the scribe school—hardly to all students, not even among those who went through the full standard curriculum, but at least to a fraction of future scribes (or future scribe school masters only?).

The word “scribe” might mislead. The scribe certainly knew to write. But the ability to calculate was just as important—originally, writing had been invented as subservient to accounting, and this subordinated function with respect to calculation remained very important. The modern colleagues of the scribe are engineers, accountants and notaries.

Therefore, it is preferable not to speak naively of “Babylonian mathematicians.” Strictly speaking, what was taught number- and quantity-wise in the scribe school should not be understood primarily as “mathematics” but rather as calculation. The scribe should be able to find the correct number, be it in his engineering function, be it as an accountant. Even problems that do not consider true practice always concern measurable magnitudes, and they always ask for a numerical answer (as we have seen). It might be more appropriate to speak of the algebra as “pure calculation” than as (unapplied and hence) “pure” mathematics. The preliminary observations on page 7 should thus be thought through once again!

That is one of the reasons that many of the problems that have no genuine root in practice none the less speak of the measurement and division of fields, of the production of bricks, of the construction of siege ramps, of purchase and sale, and of loans carrying interest. One may learn much about daily life in Babylonia (as it presented itself to the eyes of a professional scribe) through the topics spoken of in these problems, even when their mathematical substance is wholly artificial.

If we really want to find Old Babylonian “mathematicians”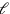 in an approximately modern sense, we must look to those who created the techniques and discovered how to construct problems that were difficult but could still be solved. 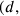For example we may think of the problem TMS XIX #2 (not included in the present book): to find the sides and of a rectangle from its area and from the area of another rectangle 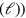(that is, a rectangle whose length is the diagonal of the first rectangle and whose width is the cube constructed on its length). This is a problem of the eighth degree. Without systematic work of theoretical character, perhaps with a starting point similar to BM 13901 #12, it would have been impossible to guess that it was bi-biquadratic (our term of course), and that it can be solved by means of a cascade of three successive quadratic equations. But this kind of theoretical work has left no written traces.

The First Purpose: Training Numerical Calculation

When following the progression of one of the algebraic texts—in particular one of the more complicated specimens—one is tempted to trust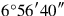 the calculations—“it is no doubt true that igi is 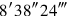, and if that was not the case, the modern edition of the text would certain have inserted a footnote” (certain writing errors have indeed been corrected above, so all calculations should be correct). The reader who has been more suspicious will, on the other hand, have received a good training in sexagesimal arithmetic.

That illustrates one of the functions of algebra in the curriculum: it provided a pretext for training the manipulation of difficult numbers. As the aim of the school was the training of professional routine, the intensive cultivation of sexagesimal arithmetic was obviously welcome.

This observation can be transferred to our own epoch and its teaching of second-degree equations. Its aim was never to assist the copying of gramophone records or CDs to a cassette tape. But the reduction of complicated equations and the ensuing solution of second-degree equations is not the worst pretext for familiarizing students with the manipulation of symbolic algebraic expressions and the insertion of numerical values in a formula; it seems to have been difficult to find alternatives of more convincing direct practical relevance—and the general understanding and flexible manipulation of algebraic formulas and the insertion of numerical values in formulas are routines which are necessary in many jobs.

The Second Purpose: Professional Pride

The acquisition of professional dexterity is certainly a valid aim, even if it is reached by indirect means. Yet that was not the only purpose of the teaching of apparently useless mathematics. Cultural or ideological functions also played a role, as shown by the “edubba texts” (above, page 33), texts that served to shape the professional pride of future scribes.

Quite a few such texts are known. They speak little of everyday routines—the ability to handle these was too elementary, in order to be justified the pride of a scribe had to be based on something more weighty. To read and write the Akkadian mother tongue in syllabic writing did not count for much. But to write Sumerian (which only other scribes would understand), that was something! To know and practice all the logograms, not least their occult and rare meanings, that would also count!

To find the area of a rectangular field from its length and width was also not suited to induce much self-respect—any bungler in the trade could do that. Even the determination of the area of a trapezium was too easy. But to find a length and a width from their sum and the area they would “hold” was already more substantial; to find them from data such as those of AO 8862 #2, or the nightmarish informations of VAT 7532—that would allow one to feel as a real scribe, as somebody who could command the respect of the non-initiates.

We have no information about Sumerian and mathematics being used for social screening of apprentice-scribes—one of the functions of such matters in the school of today: Since the scribe school was no public school with supposedly equal access for everybody, there was hardly any need to keep the “wrong” people out by indirect means. However, even in recent times dead languages have also fulfilled a cultural role beyond that of upholding a social barrier. From the Renaissance and for centuries, Latin (and “Latinity” as an emblem of elite culture) was part of the self-confidence of European administrative and juridical institutions; from that point of view, the mathematical formation of engineers was seen (by those who were in possession of Latin culture and had adopted its norms) rather as a proof of cultural and moral inferiority. Since the eighteenth century, however, mathematical competence and dexterity (at best, competence and dexterity beyond what was necessary) were essential components of the professional identity of engineers, architects and officers.¹

Even analysis of the cultural function of “advanced” Old Babylonian mathematics may thus teach us something about our own epoch.

Footnotes