1 Towards a Historical Epistemology of Space: An Introduction

1.1 The challenge of a historical epistemology of space

In the history of Western epistemological thought, there is a long tradition of dividing human knowledge into a purely rational part, independent of any experience in the outer world, and an experiential part.¹ Many aspects of spatial knowledge have traditionally been claimed to belong to the rational part. From the Pythagorean-Platonic claims about the ideal existence of geometrical figures, via early modern rationalistic ideas of deriving properties of space from pure reasoning, to the axiomatic deduction of properties of space in the logical positivism of the early twentieth century and later constructivist philosophies, attempts have been made, on very different grounds, to draw a clear-cut line between what is known of space prior to experience and what spatial knowledge is derived from experience.² Immanuel Kant’s description of space as a pure form of intuition was particularly influential. Theorems from geometry are among Kant’s prime paradigms for the existence of synthetic a priori judgments.³ In his Metaphysical Foundations of Natural Science, Kant applies his program of isolating the a priori part of knowledge to the science of his time.⁴

The historical epistemology of space is similarly concerned with identifying the different sources of spatial knowledge. At the same time it is based on a thoroughly genetic, or developmental, view of cognition. According to this view, experiential knowledge participates in the construction of cognitive structures, which in turn constitute the basis for further experience. From this viewpoint a static separation between preformed structures of cognition and contingent experiences is impossible. Or rather, it is possible only in the snapshot image of a ‘cognitive subject’. If the idea of a foundation of human knowledge – and scientific knowledge in particular – is justified, then this foundation must consequently lie in the reconstruction and analysis of the processes that have led to this knowledge over the course of time. Kant’s program of exploring which aspects of our knowledge originate in preformed cognitive structures and which aspects involve empirical insights is thus transformed into that of studying the history of the interactive processes between experience and structures of knowledge. It is in this vein that the historical epistemology of space attempts to address the problem of the epistemic status of our spatial knowledge by studying its history.

The developmental view on cognition is strongly suggested by results from different empirical disciplines. First and foremost, evolutionary biology teaches us that cognition is a function of the human organism, in particular the brain, and is therefore to be understood as a product of biological evolution. From studies in developmental psychology it has furthermore become clear that many fundamental cognitive structures are not present at the moment of a child’s birth, but are only gradually built up over the years in the long process of growing up. Finally, studies in the history of science and philosophy have revealed the historicity of fundamental concepts such as space, time, force, and matter, a historicity that became most obvious through the radical changes associated with the rise of the theories of relativity and the quantum in early twentieth-century physics.

Accordingly, we can distinguish three interwoven strands of development for which we can study the role of experience in the process of building up the perception and conception of space: 1) the phylogenetic strand, that is, the development of the biological species Homo sapiens; 2) the ontogenetic strand, that is, the development of individual human beings; and 3) the historiogenetic strand, that is, the development of human society and culture through history.

The phylogenesis of cognition is the subject matter of evolutionary epistemology. Continuity of development is produced by heredity. While experience pertains to individuals, against the background of genetic variation it shapes the development of the species stochastically through its impact on an individual’s ability to contribute its genes to the next generation’s gene pool (i.e., through selection). In this way, since the genes define a species’ cognitive potential, the experience of one generation has a bearing on the next generation’s basis for experience and thus for further cognitive evolution.⁵

The ontogenesis of cognition is the subject matter of genetic epistemology. Continuity of development is produced by the identity of an individual’s psyche. Experience may become part of the individual’s memory and may shape developing cognitive structures, which are mental reflections of real actions. The cognitive structures in turn constitute the basis for further action and related experience and, as a consequence, for further cognitive development.⁶

The historiogenesis of cognition is the subject matter of historical epistemology. Continuity of development is produced by external knowledge representations which serve the social reproduction of cognitive structures within a culture or their transfer between cultures. This reproduction relies on institutions structuring the use of the external representations.⁷ Experiential knowledge is encoded in these external representations, which in turn become the precondition for further experience and the construction of new cognitive structures. These may then become encoded in higher-order representations which are the basis for further experience and further cognitive development.⁸

The historiogenetic strand is the one that will concern us in the following pages.⁹ It is closely interwoven with the other strands in two fundamental ways. First of all, in anthropogenesis, the transition from animal to human, phylogenetic and historiogenetic factors are closely intertwined. The emergence of human culture and with it the onset of the historical development of human cognition was a result of biological evolution and, as a consequence, necessarily built upon its biological foundations. But not only did human biology condition the onset of human culture, this culture also conditioned the last steps of anthropogenesis.¹⁰

The second way historiogenesis is related to the two other strands of cognitive development is based on the fact that the species’ development, its phylogenesis as well as its historiogenesis, is realized through the ontogeneses of the individuals. Thus, the phylogenesis of cognitive structures depends on the ontogenetic transformation of the genotypes into phenotypes, and only the latter are subject to natural selection. In a similar way, the historiogenesis of cognitive structures depends on individuals who appropriate collective knowledge available in a given society at a given time in history in their ontogenesis and participate through their cognitive activities in the transmission and transformation of this knowledge.

The intertwining of the ontogenetic and historiogenetic developments of cognition explains the central role of means of external knowledge representation for understanding long-term developments in the history of knowledge. These means of representation – communicative action, spoken language, artifacts, drawings, maps, writing and other symbol systems – mediate between socially shared knowledge, which is the subject of historical development, and the individuals’ knowledge which, while being subject to all the contingencies of the individual biographies, is the only actual realization of the shared knowledge. While the external means of knowledge representation define a space of possible transformations of shared knowledge, such transformations actually occur only through the thinking of individuals, which is in turn conditioned by their participation in this knowledge.

The recognition of this dialectic between individual thinking and shared knowledge is crucial for an understanding of the aim of a historical epistemology of space as outlined here. The intention is not to provide a narrative of the world history of individual acts of spatial thinking. Such an aim would not only be unachievable, owing to the sheer magnitude of the task, but also theoretically unsatisfactory, precisely because it neglects the social dimension of thinking. The aim is rather to describe historically identifiable and theoretically interpretable cognitive configurations, or stages, that demarcate the horizon of the forms of spatial thinking that are possible in a given historical situation.¹¹

The identification of stages does not imply that the historical development of the forms of spatial thinking is a linear process. Although governed by entirely different mechanisms, this development actually shares some qualitative features with biological evolution, such as the following:

Unpredictability of future developments: Developmental processes are complex and interconnected, with the result that future developments are, as a rule, unpredictable at any time in history.

Dependency of later developments on earlier ones: Despite this indeterminacy, earlier developments produce the necessary preconditions for later ones.

Temporal directedness of overall development: This dependency of later developments on earlier ones explains aspects of the temporal order of development and makes it possible to define earlier and later stages of spatial thinking.

Asynchrony of development: The temporal directedness does not imply, however, that all development proceeds uniformly on a global scale: different stages coexist and there may even be local or temporal developments from a ‘later’ stage to an ‘earlier’ one.

In the following sections of this chapter we shall discuss six different aspects of the historical development of spatial knowledge. The similar biological constitution of all humans and the fundamental similarities in their physical environments make it plausible to assume that there are structures of spatial cognition that do not vary between different cultures or over the course of history, but constitute the foundation for all cultural manifestations of spatial knowledge (section 1.2). While similar natural conditions hold for some animal species, humans possess unique social abilities to share knowledge, a fact that constitutes the basis for the cultural evolution of human spatial cognition, leading to elaborate cultural systems for orientation (section 1.3). The transformation of human societies from bands and tribes to city states and empires created new forms of the social control of space, involving techniques of surveying, writing, and drawing, which became the precondition for the development of geometry and thereby shaped the further development of spatial thinking (section 1.4). Philosophical and mathematical texts that emerged from cultures of disputation in Greek and Chinese antiquity document theoretical reflections on spatial concepts and their ensuing generalization. Processes of reflection and generalization continued in the subsequent philosophical and mathematical traditions (section 1.5). Processes of concept formation and the generalization of spatial concepts were also promoted by the expansion of experiential spaces, be it the geographical spaces known through political expansion, trade, and exploration, be it the cosmological spaces known through observation, be it meso- and microcosmic spaces known through the integration of technical and experimental knowledge into theories of space. A prominent example is the formation of the Newtonian concept of a homogeneous, isotropic, absolute space independent of its matter content, which can be understood as resulting from reflections on an integrated corpus of mechanical and astronomical knowledge (section 1.6). The expansion of experiential knowledge about the micro and the macro cosmos and the reorganization of the knowledge of classical physics at the beginning of the twentieth century led to the demise of the Newtonian concept of space as independent from matter. According to the most advanced theory of space in present-day physics, general relativity, space and matter are inseparably related to one another. At the same time, it is as yet unclear what a theory of space for the whole of physics would look like, since the two fundamental theories of present-day physics, quantum mechanics and general relativity, disagree on basic physical concepts, such as space, time, matter, and force (section 1.7). The chapter concludes with summarizing remarks (section 1.8).

1.2 Natural conditions of spatial cognition

In order to understand how human spatial thinking depends on the cultural conditions present at different times in history it is of fundamental importance first to identify spatial abilities and corresponding cognitive structures that are not products of human culture, and accordingly not subject to historical change. These may be termed the natural conditions of spatial cognition. Starting from such an identification we may then ask how historical and present-day cultural manifestations of spatial thinking relate to this universal basis.

The natural conditions of spatial cognition have a double origin. First, there are biological predispositions of the human species which also involve a cognitive dimension. Second, there are features of the physical environment in which each individual grows up that are so fundamental that they are independent of culture. In the first case, it is the mechanisms of biological evolution by which experience enters the formation of cognitive structures, in the second it is each individual’s experience in ontogenesis. The two origins are closely entangled, since the ontogenetic unfolding of biological predispositions always takes place in a physical environment which exhibits certain universal features. While the question of the relation between the two origins will not concern us further here, it is important to note that the idea of universal aspects in human spatial cognition does not in itself imply any kind of nativism.¹²

When trying to identify the natural conditions of spatial cognition we encounter a methodological problem. Cross-cultural studies help to identify aspects of spatial thinking that are human universals, that is, aspects that do not depend on the particularities of any specific culture (for instance on the use of a particular language); yet the universal aspects identified in this manner will include aspects that depend on the very existence of human culture (for instance on the presence of language altogether). From birth (and in certain respects even before that), human beings are immersed in their culture. They are born into a cultural habitus that shapes their social and physical experiences and thus potentially exerts an influence on their cognitive development. More importantly, they participate in specifically human modes of cultural learning.¹³ As a consequence, when studying the ontogenesis of human cognition, it is practically impossible to abstract from processes of the individual’s enculturation. Therefore, to reveal its natural conditions, human spatial cognition has to be compared to animal cognition considered as the cognition of beings without human culture. Of particular interest in this context is the cognition of nonhuman primates, since cognitively they appear closest to humans and are probably similar to our not-yet-human ancestors. We could argue that natural conditions of human spatial cognition comprise their spatial abilities and the corresponding cognitive structures.¹⁴

To identify the natural conditions of spatial cognition the object of study must therefore be the spatial behavior of animals and humans (children and adults), and in particular of nonhuman primates. Of central relevance in this context are the abilities of object permanence and cognitive mapping. Let us briefly describe them. Object permanence is what developmental psychologists call the mental construction of objects as entities independent of the self, which are understood to exist in a definite location or move along a definite trajectory in space. Studies in developmental psychology suggest that what may be called the schema of the permanent object is not present at the time of a child’s birth, but only develops during the first two years of childhood.¹⁵ Object permanence skills have been proven for many animal species.¹⁶ There is thus clear evidence that the schemata of object permanence are not unique to humans. On this basis one may argue that they belong to the natural conditions of human spatial cognition.

Besides the smaller-scale skills related to object permanence, humans develop sophisticated abilities of spatial orientation on larger scales. They can quickly accumulate spatial information about previously unknown territories; in known territories they can move flexibly, that is, they can make detours and take short cuts that they have not previously made or taken; and they can optimize their routes by arranging the stations of their travel in a rational manner. They can integrate knowledge about landmarks with knowledge about the motion of their own body to construct route knowledge, and combine their knowledge about intersecting routes to obtain what may be called configurational knowledge: knowledge about the overall configuration of landmarks and their relations.¹⁷ They are also able to make use of cues such as wind directions, the position of the Sun, or distal landmarks. Following a large body of literature, we refer to these abilities here as cognitive mapping.¹⁸

Besides humans, various species of animals exhibit sophisticated performance in spatial orientation.¹⁹ Nonhuman primates in particular have been shown to be able to use spatial information in a flexible manner.²⁰ Chimpanzees, for instance, who were shown how food was hidden at several locations in a familiar environment were later able to retrieve most of the food, whereby they did not follow the order in which the food was placed, but an order that reflected a minimum-effort strategy. Using this type of strategy they could also be shown first to retrieve the kinds of food they prefer before proceeding to less favored food.²¹ Hamadryas baboons, to give another example, were being able to remember the locations of important sites such as sources of water in their local environment, using shortest distance strategies as they moved around, and even speeding up when approaching a known site well before they could have perceived it, demonstrating that they knew where they were.²²

We can summarize these findings as showing that the basic human cognitive mapping skills – similarly to object permanence skills – are not indicative of a peculiarity of human cognition but are part of its natural conditions:²³

Overall, primates have the general mammalian spatial skills of cognitive mapping and object permanence [...]. [...] It is also unlikely that humans have any special skills in these domains of spatial cognition. They too possess the general mammalian skills of cognitive mapping and object permanence [...].

Action and perception under control of the schemata of object permanence and the skills of cognitive mapping imply fundamental spatial structures which include the following:

Dichotomy of objects and spaces: Objects are tangible (albeit not always accessible), and between them there are non-tangible (i.e., ‘empty’) spaces.

Definiteness and exclusivity of place: Every object is in a place and always in one place at one particular time. No other object can be in the same place at the same time.

Three-dimensionality of objects and spaces: Objects are extended in such a way that different sides of an object are perceptible from different perspectives. Each object has a concealed reverse side. The spaces between objects are likewise extended, allowing for objects not only to be located side by side, but also to obstruct the view to another object.

Distinction of vertical direction: There is one direction determined by the tendency of most objects (including one’s own body) to fall down or to resist lifting.

Continuity of object trajectories: The mutual spatial relations of objects, including one’s own body, may change, which means there is motion. The trajectories of motions are continuous, that is, there are no ‘jumps’: objects do not vanish in one place and reappear in another, but pass through all the intermediate places during the motion. In their fully developed form, the schemata of object permanence imply continuous trajectories regardless of whether they are perceived or not.²⁴

Dichotomy of movable and unmovable objects: Some objects can be moved or move by themselves (e.g., conspecifics); other objects cannot be moved, that is, they have a fixed location (e.g., trees). These latter objects thus define a ground against which one’s own motion and the motion of other objects is perceived.

Focus on plane of movement: The space of movement (structured by a network of landmarks, places, and regions) mostly lies within a more or less horizontal plane. (The additional importance of the vertical depends on the mode of life in particular ecologies such as living on different levels of a forest, a mountainous region, or a city with multi-story buildings.)

Path-connectedness of plane of movement: The topology of the plane of movement is path-connected, that is, between any two locations there is a path connecting them (otherwise it would not be a plane of movement). Generally, there may be different paths for reaching the same location and one may travel along a closed path and come back to one’s initial location, even in cases where the path encircles insurmountable obstacles (e.g., trees, mountains, river sections, or buildings).

Dependency of effort on path taken: The effort it takes to get from one location to another generally depends on the path taken.

What is the epistemic status of the natural conditions of spatial cognition and the described cognitive structures? As we have seen, these conditions are rooted in sensorimotor intelligence, which is characterized by a close relation between cognition and concrete action.²⁵ The development of sensorimotor activity, roughly spanning the first two years of human life, ranges from reflexes via habits to the emergence of practical intelligence. In the course of this development, sensory data are assimilated to cognitive structures called schemata of action, which are in turn accommodated to the increasing amount of sensorimotor experience. The result is an increasing coordination, generalization, and differentiation of schemata of action which constitute human sensorimotor intelligence.²⁶

It is important to note that the implied spatial structures described above are not in themselves an object of thinking. They allow for successful action, but there is no indication that the related spatial abilities imply any consciousness, that is, any reflection upon the schemata controlling the actions, and thereby go beyond the sensorimotor realm.²⁷ Thus, without the dichotomy of objects and spaces, no object could be perceived or grasped. Without the dichotomy of movable and unmovable objects no stable mental representation of the environment would have been possible. Without the three-dimensionality of objects and spaces no change of the visual image could be understood as a change of perspective. But while these structures allow for spatial inferences to be drawn, they do so only in the context of action and perception and are otherwise inaccessible to the actor.²⁸ This becomes clear, for example, when school children who successfully find their way from home to school and back are unable to represent these routes in a map-like fashion.²⁹ Another example is provided by the well-attested difficulties that children have in rotating a landscape in their minds and describing how it would look from a different point of view.³⁰

In particular, there is no indication of symbol use or the dependence of spatial cognition on external knowledge representations in general.³¹ Accordingly there are also no concepts of space. The cognitive structures forming the natural conditions of spatial cognition common to all humans do not represent general, or abstract, ideas but depend on the specific contexts of action and perception. They are not to be found on the level of concepts but on that of the schemata controlling sensorimotor behavior.³²

Besides the notion of schema of action we shall employ the concept of mental model in referring to these cognitive structures. By this term we understand internal knowledge representation structures which allow current experience to be processed by relating it to former experience. The former experience is coded in the mental model in two distinct but related ways. First, the structure of the model, which consists of slots and their mutual relations, is a result of earlier accommodations to experience. The slots are filled by specific instances, that is, by an input from the current situation fulfilling certain conditions required by the slot. But these slots may also have default fillings which are effective whenever appropriate current information is not available. These default fillings result from earlier experience, thus constituting the second way in which experience is coded in the model. In this way, a mental model allows the perception of, understanding of, or even reasoning about a situation whenever the situation can be successfully assimilated to the model – even in cases where the available information is incomplete. A major reason to introduce the concept of mental model here, and not simply to speak of sensorimotor schemata, is that mental models function on different levels of cognition. The sensorimotor and practical mental models inform the models functioning on higher conceptual and theoretical levels (and these may in turn have repercussions on the lower levels).³³

The sensorimotor mental model of permanent objects is a mental structure to which sensory data are assimilated when objects are perceived and handled. For the assimilation to be successful, the shape, size, location, and position of the object must be identifiable. They do not need to be constant in time, however, although the sensorimotor schemata that underlie the model ensure that certain changes in perception are interpreted as changes of perspective, that is, of the position of the object or one’s own body in respect to it, rather than as changes of the object itself. As becomes clear from our discussion above, the sensorimotor model in its fully developed form further implies the mental representation of continuous trajectories.

To describe a range of abilities in large-scale spatial orientation, we have employed the term cognitive mapping. This term is widely used, but the precise character of the mental representation underlying the related abilities is a matter of controversy. In particular, it is not at all clear that this representation can be characterized as a bird’s eye view of the environment as the term ‘map’ suggests. Just as the mental model of object does not presuppose a three-dimensional mental image,³⁴ the mental representation of the large-scale environment need not take the form of a two-dimensional map.³⁵

Here the corresponding cognitive structures shall again be described in terms of mental models. The mental models of large-scale space may be conceived of as networks of landmarks and their spatial interrelations. It is plausible to assume that the landmarks and their relations are part of a hierarchical structure in which places and regions of different size are defined by reference to landmarks or other places and regions.³⁶ The landmarks, places, and regions are further endowed with contextual information about what is found there, e.g., kinds of food, water, predators and conspecifics, tools, and places to rest. The spatial relations between landmarks, places, and regions of different size involve topological information (inclusion, order along a route, proximity) as well as information on distances and angles. This latter information is given not in terms of numerical measures, of course, but rather in terms of sensorimotor experiences concerning variations in ease of travel, directions to landmarks, and perspectives. Configurations of landmarks, places, and regions can further be related to reference points outside the realm of motion such as the Sun or distal landmarks like a big mountain, or to overall directions defined, e.g., by a slope of the landscape or by recurring winds. The landmarks that fill the model’s slots are permanent objects or configurations of such objects, so that the elementary knowledge about objects in general (their permanence, their change of appearance with perspective and distance, etc.) applies to them. The structural relations between the slots contain the knowledge about the spatial relations among the landmarks. While the individual realizations of the mental models of large-scale space are highly dependent on the concrete features of the respective environment, since they encode the experiential knowledge accumulated as the individual moves through this environment, the basic structure applies universally. This universal structure will be referred to in the following as the landmark model of space.

1.3 Culturally shared mental models of space

If the natural conditions of human spatial cognition are similar to those of some animal species, as has been argued in the previous section, what accounts for the obvious distinction of human spatial abilities and thinking? Rather than attributing this distinction to some specifically human biological disposition for spatial cognition, the point shall be made here that the distinction can be explained as resulting from uniquely human abilities of social cognition. One argument against the existence of a specifically human module for spatial cognition is based on considerations of the necessary timescales for processes in biological evolution.³⁷ We could adduce another argument if it could be shown that the specifity of human social cognition, together with the historical development of human thought ensuing from it, can satisfactorily explain the characteristics of human spatial cognition such that no further biological factors have to be invoked. Exploring the extent to which this claim can be substantiated is a major task for a historical epistemology of space.

The human ability of social cognition implies that humans are able to communicate, to share knowledge, and to learn from each other. For this kind of cognition to arise it is crucial that humans understand their conspecifics as intentional beings, that is, as beings who act purposefully just like themselves, and are able to imagine themselves in another’s place.³⁸ In order to communicate about space, human children must learn to adopt the perspective of others. To do this they have to construct a mental representation of space that allows conception of all possible perspectives. This means the construction of what Piaget calls representational space as distinguished from perceptional space.³⁹ It is the social aspect of human cognition that implies representations that go beyond those closely tied to action and perception occurring at the latest stages of sensorimotor development.⁴⁰

Sharing knowledge crucially depends on what Piaget calls the symbolic function, that is, the ability to distinguish events and objects from their meaning. In human ontogeny this ability emerges at the preoperative stage, which succeeds the sensorimotor stage. On the basis of this ability, actions of conspecifics can be understood to mean something, that is, they become potential means of knowledge representation. Purposeful actions with the aim of communicating knowledge, like gestures, and directed joint action become possible. Tools likewise come to represent knowledge in relation to the actions performed with them. Another particularly powerful means of knowledge representation and communication is human language, which phylogenetically is assumed to have developed in the course of the Paleolithic period.⁴¹ Visual representations like drawings are also known from Paleolithic times. They are attested by various kinds of extant artifacts, most prominently the cave paintings of the Upper Paleolithic. In the course of continued cultural evolution, the means of external knowledge representation develop further themselves, for instance, under the particular socio-cultural circumstances of early city-states, when writing and the use of other sign and symbol systems such as numerical notation began to emerge (see section 1.4).

Consequently, the crucial distinction between animal and human cognition is the emergence of a cumulatively evolving human culture, a thoroughly social phenomenon. For every ability of individual humans that may be argued to play a crucial role in the emergence of this culture, such as the ability to use and produce tools, or to understand conspecifics as intentional beings, or to understand symbols and develop language, we find precursors in the animal kingdom.⁴² Rather than being attributable to a single distinguishing factor, the animal-human divide seems to emerge from a process in which social, material, and cognitive developments interact in a complex causal structure.⁴³

An immediate consequence of the cultural evolution of human societies for spatial cognition is that the mental models of large-scale space become culturally shared. In addition to those commonalities between two individuals’ mental models of space that are due to their similar biological constitutions and their similar experiences within the same environment, human mental models of space display cultural commonalities. In this way the mental models of space themselves become part of an evolving culture, accumulating collective experience over generations and becoming richer and more refined than any mental model a single individual could have produced.

The sharing of mental models of space appears to be common to all human societies, from nomadic tribes to modern urban societies. When considering the impact of the cultural sharing of knowledge on the mental models of large-scale space, the general objects of study are therefore the practices of navigation and spatial orientation and their externalizations in language and other artifacts in all kinds of human societies. In most contemporary societies, however, these practices involve specialized means of spatial representation and advanced technology which have developed over the long course of history. To get an idea of what can be achieved in the absence of maps, compasses, sextants, or GPS receivers, we have to study the spatial practices of nonliterate societies that do not employ such specialized material tools. In the case of prehistoric societies, the archaeological evidence is the only available source for a reconstruction of such practices. In the case of recent nonliterate societies, by contrast, spatial practices, including their spatial language, can be investigated much more directly, which makes them an advantageous object of study.

Recent nonliterate societies show a wide variety of cultural systems for spatial orientation and communication.⁴⁴ This cultural diversity is due not only to the self-referential dynamics of cultural evolution, but obviously also to the fact that these systems represent responses to the challenges of widely differing ecologies to which they are adapted. Nevertheless, there are common patterns that may be discerned. It may be observed, for instance, that toponyms play a central role in spatial reference in a wide range of societies. Places and their relations are richly endowed with meanings relating to mythology, the history of places, and the natural knowledge about them. In many societies this practice is additionally complemented by a system of absolute directions, which in some cases plays such a crucial role that members learn always to keep track of these directions.⁴⁵

Two examples of recent nonliterate societies and their spatial language and practices are discussed in this book, the Eipo living in the central highlands of West New Guinea, and the Dene Chipewyan living in the Cold Lake region in Alberta, Canada (Chapter 2). The spatial knowledge described in this context may be characterized as practical knowledge. Among its characteristic features are: its transmission through external knowledge representations; its cultural organization; its dependence on the specific contexts of action; and its locality.

Transmission through external knowledge representations. In contrast to sensorimotor knowledge, which is built up in the individual’s interaction with the physical world, practical knowledge is built up through social interaction and communication. The knowledge representations employed in this context include joint activity and action with the explicit aim of teaching, the tools and artifacts produced through such actions, and spoken language. While the communication builds upon shared sensorimotor structures, the use of external knowledge representations makes it possible to accumulate knowledge that could never be acquired solely through one individual’s experience.

Cultural organization. This accumulation is accompanied by the cultural organization of knowledge – which, in fact, makes the mastery of the accumulated knowledge possible in the first place. Culturally shared large-scale space is spanned not only by landmarks, places, regions, and their relations, but by the meanings attached to these entities. These meanings organize the spatial knowledge and are given in form of nomenclatures, narratives (mythical or otherwise), or sets of practices. Place and spatial order play an important role in Eipo myths, for instance, and, conversely, mythical narratives are instrumental in handing down spatial knowledge.⁴⁶ In contrast to the sensorimotor mental models of space, large parts of this mental representation may be accessed deliberately by its holder, particularly in order to communicate about space. Besides the cognitive dimension, the cultural organization of knowledge further implies an institutional dimension: The social reproduction of knowledge relies on more or less stable social patterns (institutions) structuring the collective use of the means of knowledge representation.

Dependence on the specific contexts of action. The spatial concepts structuring practical knowledge are, as a rule, not abstract or general but depend on the specific contexts of action. They are not applications of more general concepts in concrete situations but are rather conditioned by these situations. Further, the way in which the concepts structuring practical thinking about large-scale space relate to more small-scale spaces remains largely undefined. As a consequence, metrization remains fragmentary. Distances measured in terms of days of travel are not brought into any relation with cubits or other measurements of length which may be employed on a different scale.

Locality. The shared mental models of large-scale space are local in character. Practical concepts of space depend on the particular features that make up the space, and are not generally applicable to arbitrary environments. Systems of toponyms, for instance, obviously apply only locally, since they inherit the dependence on the particular environment from the landmarks and relations they refer to. The same holds for most variable cues such as winds or swell-patterns. But more structural elements of the system of orientation may be dependent on local peculiarities as well. Thus, the widespread use of star positions for determining directions by Micronesian expert navigators only works due to the proximity of their islands to the equator, since it is only there that the stars and constellations rise and set nearly perpendicularly to the horizon.⁴⁷

To sum up, culturally shared mental models of large-scale space may be understood as collective elaborations and modifications of sensorimotor models. Just like the latter they are based on the landmark model of space, from which they inherit many structural features. At the same time, they encode a larger body of experiential knowledge than the sensorimotor models: they integrate the experiential knowledge about the environment not only of one individual but also of whole societies over the course of many generations. This integration is achieved by means of the cultural organization of knowledge, which necessarily reflects features of the local environment and displays cultural characteristics. Elementary knowledge structures thus serve as a foundation for culturally shared practices without determining their cognitive dimension. At the same time, culturally transmitted knowledge has repercussions on the more elementary level of sensorimotor knowledge when action and perception based on a culturally shared mental model of space becomes intuitive.

1.4 Social control of space and metrization

An immediate consequence of the cultural evolution of human societies on spatial cognition, which was discussed in the previous section, is the development of elaborate practices of spatial orientation based on shared mental models of large-scale space. Another way in which the cultural evolution of human societies shapes spatial thinking is based on the fact that the organization of society implies the social control of space. How is space divided among different individuals and social groups, what is the social function of different places, what are the places for public, sacred, or private affairs, who is allowed to go where, and who is allowed to use what land or even owns it? Questions of this kind can be observed to arise in the context of the organization of any human society.

The means for the social control of space depend on the respective form of social organization. In the case of small rural communities such as that of the Eipo described in Chapter 2, we may speak of the mythical control of space. Under the mythical control of space, knowledge about the social function of different places and about the allocation of space is largely represented by myths, which also ensure its social implementation. Despite the central role the division of land plays in social life, the mythical control of space does not provide standardized tools for measuring lengths and distances or for determining the quantitive measurement of an area. The Eipo’s construction of a sacred men’s house of defined size and shape, for example, is a complex task which is mastered without recourse to material representations of spatial knowledge such as measuring rods, drawings, or any kind of specialized geometric language. Instead, the spatial knowledge necessary to build the house is embodied in the ritual actions specific to the Eipo culture.⁴⁸ The distribution of garden lands among the Eipo is governed by clan-membership, heredity, and the capacity to cultivate the land. There are practices for delimiting fields (the demarcation of land by sacred Cordyline trees), but not for determining or estimating field sizes. Conflicts over the right to use a piece of land may lead to hostilities or be solved by negotiation, but their resolution never involves measurement.⁴⁹

Historically, the earliest evidence for the systematic use of standardized measures for the social control of space stems from the so-called early civilizations. The growth in population of neolithic sedentary communities in some areas of the world went along with the development of increasingly specialized food production, irrigation, and food storage technologies, and resulted in the emergence of stratified societies that controlled progressively larger spaces. The formation of city states and larger empires brought about new phenomena in human culture such as centralized administration, property regimes, monumental architecture, centralized religion, and new forms of standardized means of knowledge representation. In particular, it gave rise to new forms of the social control of space which may be referred to as the administrative control of space. These forms involved techniques of measuring, surveying, writing, and drawing, which implied a progressive metrization of space and led to a kind of proto-geometry.

A decisive strand in this bundle of developments was the emergence of new forms of the division of labor. Besides gender-specific forms of division of labor (with hunting considered as a predominantly male activity, for instance) or practice-specific forms (as in the case of the experts of Micronesian navigation), a fundamental social division became manifest: the division of physical and intellectual forms of labor. In general we can discern a physical and an intellectual component in the human practices of using and producing tools. Concrete action is preceded by planning, that is, selecting tools, determining the sequence in which they are used, and coordinating work in cases where more than one individual is involved. The growing complexity of the planning and organizational tasks in the stratified societies of the early civilizations led to a division of labor along this intellectual-physical divide. The result was a specialization of intellectual labor which became manifest in the emergence of professions such as the scribe, the administrator, and the surveyor, and in an administrative hierarchy reflecting the emergence of mental activities that coordinated other mental activities.⁵⁰

As these mental activities are themselves dependent on material tools, the development of early civilizations went along with fundamental innovations in the means of external knowledge representation. This holds in particular for activities related to the social control of space such as architecture, urban planning, surveying, and field measurement which involved means of semantic and numerical notation as well as tools for graphical representation such as the compass and the ruler. Among the early civilizations in which such techniques developed are those of Mesopotamia, Egypt, China, and Mesoamerica and South America. The developments are well documented in the case of Mesopotamia, where proto-writing emerged before 3000 BCE on the durable medium of cuneiform tablets so that a large amount of administrative records are preserved. Evidence for similar developments in other early civilizations is more indirect. In the Egyptian case we have depictions of surveyors at work, e.g., the wall painting in the tomb of Menna in Thebes,⁵¹ and mathematical texts on the calculation of areas such as parts of the Rhind Papyrus, but no administrative documents on the determination of field areas have been preserved. Evidence in the Chinese case comes from much later periods and again does not document early administrative practices.⁵²

In this book the emergence and early development of the administrative control of space, and the related gradual metrization of space, is discussed using the example of Mesopotamia (Chapter 3). The history, ranging from early Mesopotamian practices of surveying to Babylonian geometry, spans millennia in which fundamental developments occurred, such as the invention of the sexagesimal place value number system.⁵³ Accordingly, the spatial knowledge discussed in Chapter 3 ranges from practical knowledge to mathematical knowledge. It is the expert knowledge of a particular group of administrators and develops over history along with the means of symbolic representation. It is externally represented by measurement devices, drawings, and symbolic notation, which develops into writing on one hand and numerical notation on the other.⁵⁴ It thereby reproduces structures found on a more elementary level of cognition, this time, however, endowing spatial entities with arithmetic properties. This arithmetization of spatial entities also leads to an integration of spatial structures which remain separated on a more elementary level. Let us give two examples.

The conservation of the size and shape of an object independent of its location and position is implied by the sensorimotor schemata responsible for the coordination of perspectives. It is further implicit in the comparison of the size of objects by means of juxtaposition when no standardized means of measurement are available. The assumption of the conservation of the size of an object when it is moved through space is, in fact, a precondition for the use of measuring rods or ropes. In the context of the use of such tools and in the presence of standard measures of length, area, and volume, the conservation of size becomes manifest on the level of mathematical representation and implies metric homogeneity of space. This arithmetization also serves as a precondition for the integration of spaces of different orders of magnitude through the coordination of the units of measurements on different scales.

The three-dimensionality of objects and spaces is another example of the integration of spatial structures through arithmetization. Three-dimensionality is perceptually given on the sensorimotor level. Through the arithmetical dependencies between length, area, and volume it is reproduced on the level of the symbolic means of knowledge representation and enables the reflection on the relations between entities of different dimensionality.

The metric structure of space becomes more generalized through the application of the sexagesimal place value number system with its general procedures for addition, subtraction, multiplication, and division, and in combination with an abstract system of units defined by its internal relations. This illustrates how, in certain historical situations, the emergence of new means of knowledge representation in specialized practical contexts (surveying) may lead to a dynamic of knowledge development that engenders knowledge structures no longer directly related to that context (Babylonian geometry). But this greater generality implicit in the symbolic means of knowledge representation must not necessarily be made explicit, for instance, in the form of a term that represents the concept of a three-dimensional metric space spanning various scales.

Despite its novel degree of abstraction and its thorough metrization of area, the Babylonian surveyors’ 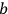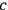mental model of space actually differed from Euclidean space. The procedures of Babylonian geometry are of a limited generality which testifies to their origin in administrative practices. In particular, there is the striking absence of the consideration of angles as objects of mensuration, which is rooted in the implicit definition of area by means of what is known as the surveyors’ formula, that is, the rules of calculation for determining the area of irregular quadrangles of sides , , , and 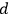, which corresponds to an application of the formula 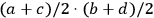. Field areas are thus calculated on the basis of lengths without quantitatively accounting for the angles.⁵⁵

1.5 Context-independence of mental models resulting from reflection

The cultural developments of spatial thinking discussed in the preceding section show a basic trend towards cognitive structures that are less dependent on the specific practical contexts from which they originated. An example is the emerging practice of area determination by means of a multiplication of lengths within the sexagesimal place value system, which implies a greater degree of generality than any conventional way of relating areas to standard lengths based on specific practices of measurement and notation. The increase in generality is obviously related to the development of the means of knowledge representation such as comprehensive systems of units and a place-value number system. But this development is only the material side of a dialectical process whose other side is mental. Performing operations on external knowledge representations builds up structures which are mental reflections of these operations. Since these operations disregard many aspects of the real-world objects, this mental process may be referred to as a reflective abstraction. When the new mental structures are in turn externally represented, e.g., by symbols forming a system, we may speak of a representation of higher order than the one from which the process of reflection started.⁵⁶

Processes of reflective abstraction are a consequence of the exploration of existing means of knowledge representation. Exploration of these means by individuals may happen spontaneously at any time in history. But such individual developments remain without consequences in the history of knowledge unless there are social entities such as organized groups or institutions that ensure that the cognitive products are handed down and – at least for a certain period – become subject to cumulative development. A potential case of this type of institutionalization are the schools of the scribes in Mesopotamia which developed Babylonian geometry as a doctrine of areas independent of the context of surveying – even though the structure of Babylonian geometry still bears witness to its origin in practical surveying (as argued in the previous section; see Chapter 3). The context of teaching and learning the handling of symbolic means of knowledge representation seems to be a natural place for the emergence of exploratory forms of knowledge. Another such context is disputation, traditions of controversial discourse and rational debate. While such traditions are usually oral in origin, they may find expression in text traditions, possibly accompanied by an ongoing oral component. Disputation is a motor for reflection on concepts and, as a consequence, for their generalization. The resolution of apparent paradoxes, for instance, presupposes reflection on language and the delineation of meanings. Spatial knowledge need not be the primary object of these reflections, but if the aim is comprehensiveness it will naturally come into consideration.

One may distinguish two types of explorative knowledge, which may roughly be designated mathematical and philosophical. Mathematical explorative knowledge results from systematic reflection specifically on representations related to the use of instruments such as measuring rods and ropes, the straight edge, and the compass.⁵⁷ Philosophical explorative knowledge, by contrast, results primarily from systematic reflection upon the linguistic representations of elementary shared knowledge.

Among the most prominent historical settings in which the exploration of the cognitive tools of spatial thinking became productive are the intellectual traditions of ancient Greece. The first-order knowledge that was reflected upon in this context was by no means of purely Greek origin. From the Archaic period on, astronomical, medical, and arithmetical knowledge from Egypt and Mesopotamia entered the Greek world.⁵⁸ In contrast to the Babylonian case, which was defined by the needs of central state administrations, the Greek situation was characterized by polycentrism, the encounter of different strata of society, and the negotiation and public justification of political decisions.⁵⁹ This was the background for pursuing systematic reflections which aimed at establishing a coherent, encompassing world view, distinct from the received mythology but with the same aspiration to totality. Written texts produced in the context of the Greek philosophers’ activities now provide us with the earliest evidence of systematic reflections on the linguistic representation of shared spatial knowledge. A parallel and related development is the formation of a characteristic Greek tradition of mathematics, particularly concerned with questions of geometry.⁶⁰

Among the later historical intellectual places which furthered deliberate and purposeful exploration of the implications of systems of knowledge representation were the Neoplatonic schools of late antiquity, Hellenistic science as pursued at the Museion of Alexandria, court science, philosophy and theology of the Arab Middle Ages as pursued in Bagdad and Córdoba, and the scholasticism of the Latin Middle Ages. In early modern times the theoretical reflection on fundamental concepts such as space and matter gained new impetus in the context of an ideological struggle between different strata of society. In their attempts to formulate encompassing counter world systems against the predominantly Aristotelian world view promoted by the Church, early modern natural philosophers faced the challenge of taking account of an increasing amount of empirical knowledge from practical mathematics and astronomy.⁶¹ In the following centuries, theoretical reflection on space has become increasingly institutionalized in the disciplinary discourses of physics and philosophy.

All of the historical periods and places mentioned so far, in which the exploration of and reflection on representations of spatial knowledge took place, are more or less strongly related by ties of tradition: they all, in one way or another, relate back to the theoretical traditions of Greek antiquity. The example discussed in greater detail in this book, by contrast, presents a rare case of independent emergence of systematic reflections on spatial language; it is documented in the so-called Mohist Canon, a text from Warring States China, ca. 300 BCE (Chapter 4). The Mohist reflection clearly represents what we have referred to above as philosophical explorative knowledge, the systematic reflection on the linguistic representations of elementary knowledge, although references to mathematical instruments are also found in the text. Compared to the Greek case, the Mohist Canon represents a unique source for addressing comparative questions in the long-term history of spatial knowledge; questions concerning the conditions for the emergence of traditions of systematic reflection and the necessities and contingencies in their development.

The spatial knowledge considered here can be described as theoretical knowledge. This kind of knowledge is largely conditioned by its means, that is, by the external knowledge representations from the exploration of which it emerges. It is handed down in text traditions, mostly in form of written language and symbolic notation, which make it possible to pick up a tradition again even centuries after it has last been actively pursued (although the case of the Mohist Canon shows that it may also be handed down without ever having been actively taken up again). It is aimed at consistency and comprehensiveness and thereby gives rise to more general and abstract concepts such as those of Euclidean distance and the atomistic absolute void, sometimes including a general concept of space.

The explorative reflection upon elementary structures of spatial thinking creates theoretical structures which preserve many of the spatial properties implied by sensorimotor intelligence. At the same time, the theoretical context of generalization and aspiration toward consistency leads to questions about these properties which could never have occurred in elementary or practical contexts. At the level of fully developed sensorimotor activity, the mental models have their clear-cut realm of applicability. At the level of theoretical thinking, by contrast, there is an inherent uncertainty about what aspects of the mental models to build upon. This ambiguity derives from the absence of the concrete contexts of action that limit the meaning of the linguistic representations of knowledge in their everyday use. The operations on external representations in reflective thinking are dissociated from these original contexts and produce structures inherent in the system of representations. The result of such processes of reflective abstraction are not predetermined in general, because the space of possible structures spanned by the means of representation is much richer than any particular realization in it. The analysis of the Mohist passages and their comparison to Western sources in Chapter 4 shows, among other things, that the occurrence of elementary mental models in theoretical thinking on space is indeed a cross-cultural phenomenon. The connection of such reflections with encompassing worldviews, by contrast, is a peculiarity of the Greek case and depends on the timing of specific theoretical traditions such as the construction of cosmologies on the one hand and the reflection on the meaning of words on the other.

There is a striking difference between philosophical and mathematical explorative knowledge. While the former depends on individual decisions motivated within more encompassing knowledge systems and remained controversial throughout the history of philosophical thinking, the latter was, from early on, considered to present inevitable truths. The well-defined object of reflection of mathematical explorative knowledge, the first-order representations related to the use of instruments (figures drawn by means of a straight edge and compass in the case of Euclidean geometry), allowed for a consistent representation within a deductive structure. The reflection on first-order representations thus led to a generalization of spatial concepts which implied a de-contextualization: what had been a theory of constructed figures became interpreted as a theory of space, decoupled from what fills space.⁶²

The reflection on the higher-order representations of Euclidean geometry (deductively organized sets of statements) further generalized the spatial concepts when the possibility of non-Euclidean geometries was discovered. It thereby led to theoretical alternatives in the case of mathematical knowledge as well, theoretical alternatives which could not be evaluated on purely rational grounds. As a consequence, it led to a re-contextualization of geometry, because there was a new appreciation of the role of rigid bodies (and light rays) for establishing the geometry of physical space. The emergence of non-Euclidean geometries thus functioned as a historical reminder of the empirical origins of Euclidean geometry in instrumental action. Accordingly, and in spite of deviating epistemological claims, the question of the applicability of non-Euclidean geometries was revealed as an empirical question. In this context, first-order representations of spatial knowledge (measuring rods), became higher-order representations that relate abstract structures to physical space by connecting theoretical knowledge with other layers of knowledge.⁶³

1.6 The expansion of experiential spaces over history

In the previous section we argued that reflection on the external representations of elementary and practical knowledge may lead to new and more general spatial concepts. In such cases of theoretical thinking, novelty arises from the structures inherent in the means of knowledge representation and tools for intellectual labor becoming explicit through being explored and through reflective abstraction. But the history of theoretical reflection does not unfold before a background of unchanging spatial experience. If we are concerned with the relation of experience and theoretical reflection in the historical development of spatial concepts, we have to take into account a complementary long-term trend: the expansion of experiential spaces. This expansion of experience not only implies an accumulation of spatial knowledge but also plays an important role in creating new spatial concepts and stabilizing them within more comprehensive knowledge systems.

Starting with the first steps of ontogenesis, experience plays an instrumental role in shaping human spatial cognition (section 1.2). Beyond the immediate experiential environment of the individual, different socially shared spaces can be experienced in different societies. This experiential basis of spatial knowledge expanded in the course of history, not monotonically and not universally, but within a long-term, global perspective. One may distinguish three realms of experiential space to which this expansion pertains. First of all it pertains quite literally to the geographic spaces known to human societies, which have grown through travel, trade, exploration, and military campaigns. Such activities led to the expansion of the space for movement of various societies or even of their organized space, as in the case of expanding empires which take political and economic control of more and more territory. These spaces have grown in many local historical contexts and in a long-term perspective, spanning the time from prehistoric nomadic and sedentary tribes to modern global societies that enable intercontinental travel and communication.

Another experiential space that has expanded over history is cosmological space. Cosmological space is the entire universe known, or assumed to exist, by a given society. Society transfers spatial concepts and knowledge acquired in terrestrial contexts to this space. It is, in particular, also the space of mythical realms of experience. Cosmological space is experiential through the observation of the sky, especially systematic astronomical observation. This space has grown enormously, from observations of the Sun, the Moon, the planets, and the stars in early societies, to the modern observation of astronomical objects billions of light years away. It has also grown with respect to its wealth of physical contents. With the increasing refinement of celestial mechanics from antiquity to modern times, and with the rise of astrophysics in the course of the nineteenth and twentieth centuries – developments clearly related to the progress of observational instruments and techniques – the import of knowledge from terrestrial science into cosmology has vastly increased. With the observation of the flight of the galaxies, cosmological space itself has been turned into an object to which elements of physical description, such as the field equations of general relativity or the model of a black body, may be applied. Visible light has become just one of a wide range of sources for knowledge about the universe, and present-day astronomy is reaching the brink of the observable universe: looking far away means looking back in time, and with the most recent breakthrough in the detection of gravitational waves⁶⁴ there is the justified expectation that we will soon be able to ‘look through’ the early universe which is opaque with respect to electromagnetic radiation.

Microcosmic space, just like macrocosmic space, has been a target for projection of experiential knowledge from the mesocosmic realm, as the example of atomism discussed in the previous section illustrates. On the background of such theoretical world views, knowledge about physical objects acquired through practical experiences in dealing with technological artifacts or even through systematic experimentation has potential implications for spatial concepts. The expansion of experiential knowledge about the micro-world was not only due to new instruments of magnification – from the optical microscope to the particle accelerator – but also to the systematic exploration of chemical, electric, and magnetic phenomena. In particular the increase, in modern times, of empirical knowledge in the fields of mechanics and electrodynamics led to fundamental changes in the concept of space, the first being related to Newtonian absolute space, the second to the spacetime of special relativity.

When considering the impact of the expansion of experiential spaces on spatial thinking, the objects of study are processes of concept formation fostered by the increase of experiential knowledge in the three realms described above: geographical, cosmological, and microcosmic space. Two examples are discussed in the present book: the geographical and cosmological knowledge on which the insight into the spherical shape of the Earth and the idea of its central position in a spherical universe are based, and the different ways to argue for this idea that are found in Aristotle and Ptolemy (Chapter 5); and the transformation of natural philosophical considerations on the relation between matter and space through the growth of the corpus of empirical knowledge on mechanics and astronomy (Chapter 6).

The knowledge discussed in these chapters is once again theoretical knowledge. Unlike the knowledge discussed in the previous section, it is theoretical knowledge resulting from systematic extensions of its experiential base. The accumulation of experiential knowledge takes place within institutions specifically designed for the purpose of knowledge acquisition⁶⁵ and often occurs using instruments specifically designed for the purpose of knowledge acquisition such as astronomical instruments and laboratory equipment. The empirical knowledge is organized in integrative structures based on symbolic and formalistic tools such as numerical coordinates, analytic geometry, calculus, and differential equations. The way the symbolic tools are used is shaped by the experiential knowledge to be integrated. At the same time, the symbolic tools are related to concepts and have a repercussion on conceptual structures. It is via the interaction of experience, symbolic representation, and concepts that experiential knowledge shapes conceptual structures. In this process of reflection upon the institutionally accumulated empirical knowledge, the mental models, which were based on elementary and practical experience, are transformed. The accumulating knowledge and its symbolical-formal integration thereby produce and stabilize models and concepts that are highly counter-intuitive. Examples of such counter-intuitive knowledge structures are:

The Earth has a spherical shape (cf. Chapter 5). The idea of a spherical Earth violates the distinction of the vertical direction in elementary spatial cognition.

Matter is nothing but empty space permeated by forces (cf. Chapter 6). This idea (formulated by Kant in his Metaphysical Foundations of Natural Science) violates the dichotomy of objects and space in elementary spatial cognition. In a certain sense it anticipates the later field concept that emerged in nineteenth-century research on electromagnetism, a concept that represents a hybrid of bodily and spatial properties.

The theoretical knowledge resulting from the expansion of experiential spaces has repercussions on different layers of knowledge. Global, geographical coordinates, for instance, attained practical importance in deep-sea navigation. Coastal shipping primarily relies on landmarks. Mediterranean seafaring from the late Middle Ages on could use the magnetic compass complemented by portolan maps displaying compass directions and distances. But for deep-sea navigation knowing one’s absolute position is crucial, since in vast regions there are no landmarks and the distances are too large for dead reckoning. After the discovery of electromagnetic radiation, radio navigation became an important tool for spatial orientation at sea.

Theoretical knowledge resulting from the expansion of experiential spaces also has repercussions on theoretical knowledge in general. The insight into the sphericity of the Earth, for instance, which was stabilized by the expanding geographical knowledge, had far-reaching consequences for theories of space, as its central role in Aristotelian physics and cosmology illustrates. The success of electrodynamics, to give another example, inspired the electromagnetic worldview which held that all matter should be reducible to fields. Further, the application of the field model to gravitation lay at the foundation of the development of general relativity, as will be discussed in the following section.

But theoretical knowledge resulting from the expansion of experiential spaces may also have an impact on meta-theoretical knowledge. This is strikingly demonstrated by the influence of Newton’s concept of space on Kant’s epistemology. Long before writing the Critique of Pure Reason, Kant had read the Leibniz-Clarke correspondence and occupied himself with the concept of space, considering aspects of Leibniz’s as well as of Newton’s conceptions. In the Critique, Kant presents space as the pure form of outer intuition and states that⁶⁶

[w]e can never have a presentation of there being no space, even though we are quite able to think of there being no objects encountered in it.

While space is thus a precondition of experience, rather than being derivable from experience, matter is not so, as Kant explains in his post-critical Metaphysical Foundations of Natural Science, in which he endeavors to provide a sound metaphysical foundation for Newtonian mechanics. In contrast to space, matter is an ‘empirical concept’, that is, it requires perceptually given instances in order to attain objective reality.⁶⁷ This epistemic divide between space and matter was not part of Newton’s philosophy of space. But it was only the autonomy of Newton’s concept of space with respect to the concepts of things in space (matter, force) that made Kant’s epistemic separation possible. Kant clearly argues on the basis of a container model of space,⁶⁸ even though he does not argue for the reality of this container but only for its necessity in cognition.⁶⁹ Kant’s epistemic separation of space and matter would not have been possible against the background of Aristotelian physics or general relativity, both representing frameworks in which space is (in very different ways) inseparably intertwined with matter.

1.7 The decline of an autonomous concept of space

In the previous sections we have argued that more and more general concepts of space emerged under more and more specific cultural conditions. In societies where centralized state administrations took over the social control of space, spatial measures became more standardized and integrated and eventually assumed general arithmetic properties (section 1.4). In societies where oral and written disputation became a social practice, spatial terms formerly used in the context of specific contexts of action attained abstract meanings defined by their position in more encompassing conceptual systems (section 1.5). Under specific historical circumstances in early modern Europe, the integration of different historical strands of knowledge culminated in Newtonian mechanics and gave rise to a concept of space that was not only general but, at the same time, implied the autonomy of space from other physical entities represented by fundamental concepts such as matter, force, and time (section 1.6). With regard to its autonomy the space of this conception was similar to the void of ancient atomism, yet it was clearly not conceived of as nothing, but rather as a physical entity in its own right, sometimes even as a substance, and often as conceptually prior to the things filling space.

The trend for increasingly general spatial concepts under ever more specific cultural conditions did not continue, however, within institutionalized physics and its neighboring disciplines over the course of the twentieth century. It is true that the concepts of space employed in modern physics are more general than the Newtonian concept in that they pertain to theories that are able to integrate a larger corpus of empirical knowledge. We can give an obvious illustration of this fact by referring to general relativity, which contains Newtonian gravitation theory as a limiting case and, in addition, is able not only to predict the advancement of the planets’ perihelia as well as the bending of light by gravitation with high precision, but also to describe the spacetime dynamics of massive objects such as galaxy nuclei and, in fact, of the universe in its entirety. Yet, in two important respects the Newtonian concept constitutes the historical acme of the generality of concepts of space: it was thought of as fundamental not only for the theory of mechanics from which it arose, but for the physical world in general, regardless of what was considered to be in that space and what discipline described things in space. It was further considered to be universal in the sense that space was the same everywhere: it was homogeneous and isotropic. This property was closely related to its autonomy from other fundamental concepts; since the distribution of things in space (matter and forces, say) is obviously not homogeneous, space has to be decoupled from these things in order to be so.

In twentieth-century physics these two aspects of generality became inapplicable to the developing concepts of space. While the aspiration of formulating fundamental concepts underlying all of physics has always remained a part of the agenda of theoretical physics, and unification is one of the major challenges of present-day theoretical work, there is no concept of space in twentieth-century physics that could consistently be applied to all fields of physics. The same applies to the concepts of time, matter and force. The most advanced concept of space in a well-established theory of modern physics is clearly that contained in the dynamic spacetime of general relativity, which also plays a central role in modern cosmology. At the same time, this concept of spacetime is not compatible with quantum theory, which has so far provided us with the most advanced theory of matter and radiation. Thus, quantum field theory usually presupposes a special-relativistic spacetime, and quantum mechanics is mostly done in non-relativistic space. It is unproblematic, of course, to understand fundamental concepts such as matter and space differently in the different fields of physics. The point is that, if these different usages are understood as resulting from the consideration of limiting cases to a unifying theory,⁷⁰ such a unifying theory has not yet been established and we do not know what its concept of space will look like. There is not even agreement on the way the two fundamental theories of twentieth-century physics, quantum theory and general relativity, are to be combined for an advanced understanding of their relation. Is quantizing general relativity the solution? Or, on the other hand, can gravitation theory explain quantum mechanical measurement?⁷¹

The autonomy of space, its independence from time, matter, force, and motion, which was a precondition for its universal homogeneity and isotropy, is lost in twentieth-century physics, too. With special relativity, space becomes entangled with time in such a way that their separation depends on the relative state of motion of the observer and the system under consideration. With general relativity, this spacetime becomes further entangled with matter and force; where the geometry of spacetime is determined by matter (and other forms of energy), and determines the motion of matter and radiation under what was classically considered the gravitational force. Spacetime and matter are entangled so closely that a consideration of the two separately (what is the geometry of spacetime? – How is matter distributed in that spacetime?) can only be done in special cases and only approximatively, while the full theory always demands consideration of both at the same time. Quantum theory provides further intriguing instances of an intertwining of spatial and material concepts, as may be illustrated by reference to non-local phenomena such as quantum entanglement. But however radical the changes quantum theory has effected with respect to the concepts of matter and radiation, it has not (yet) led to a new concept of space. In this book the discussion relating to the decline of an autonomous concept of space is focused on the question of which parts of the experiential knowledge of modern physics had an impact on the concept of space and which parts did not, and how this disparity can be explained (Chapter 7).

The spatial knowledge under discussion in this context is a particular kind of theoretical knowledge, knowledge that develops only in a science that is highly structured in terms of disciplines and sub-disciplines.⁷² This knowledge is characterized by a hierarchy of divisions into areas that display specific knowledge structures comprising area-specific concepts, models, and methods. At the same time, different areas are connected by the overlap of certain concepts, models, and methods. Particularly, fundamental concepts such as space, time, energy, matter, and force relate different areas, without necessarily being understood in the same way in every area. Areas may further be connected by objects of study whose treatment requires specific knowledge from more than one area. The knowledge structures within these areas are comparatively stable over periods of time, but knowledge integration across area-boundaries leads to fundamental changes of structure.

The boundaries between the areas shift in various ways over the course of time, resulting in knowledge integration and disintegration, but overarching theories remain a challenge. Thus, the theory of special relativity resulted from the integration of mechanics and electrodynamics into a unified spacetime framework. This led to the temporary disintegration of gravitation, which had formerly been a part of mechanics. The re-integration of gravitation, mechanics and electrodynamics in a unified spacetime framework resulted in the development of general relativity. Quantum mechanics, which had emerged from the consideration of problems on the boundary between thermodynamics and electrodynamics, and further integrated knowledge from mechanics, was faced with the challenge of integrating relativistic field theory. The integration of special-relativistic electrodynamics into a quantum framework – quantum electrodynamics – left the gravitational force – general relativity – standing alone again.⁷³ In this sub-disciplinary landscape, the two theories of relativity play quite different roles. Special relativity provides the spacetime framework for a large number of sub-disciplinary fields, while general relativity, albeit the more fundamental theory, is comparatively isolated.

The knowledge organized according to disciplines is represented by means of highly specialized technical languages, often employing symbol systems, in particular mathematical formalisms. Empirical knowledge is systematically produced in various subfields. The knowledge resources in their disciplinary configuration define a space of possible transformations and thereby condition the outcome. This means that even in cases in which, historically, the development relies on the particular contribution of a single individual, the configuration of knowledge conditions the outcome of the transformation. In particular the invention of general relativity may appear as contingent on Einstein’s peculiar insistence on the incorporation of the equivalence principle in a relativistic theory of gravitation, and his isolated work ensuing from it. Nevertheless, granting the necessity of consolidating gravitation with relativity, and given the knowledge resources of classical mechanics, one is almost inevitably led to spacetime curvature. Thus, despite Gunnar Nordström’s more conservative approach to a relativistic theory of gravitation, his final theory exhibits a curved spacetime, as could later be shown.⁷⁴ One can advance very basic arguments, requiring energy conservation, deriving the equivalence principle from it, and then showing that in special relativity this inevitably leads to curved spacetime geometry.⁷⁵ Theories with a tensor potential starting off in a flat Minkowski spacetime also turn out to exhibit a curved spacetime, once their inconsistencies are eliminated.⁷⁶ One may thus conceive of very different historical pathways, probably distributing innovative contributions across more individuals, and combining the classical resources in different temporal order, all eventually leading to a theory very similar to general relativity – or maybe, much less probably, directly to a theory of quantum gravity!⁷⁷

1.8 Concluding remarks

This introduction started by raising questions about the epistemic status of spatial cognition. What is the relation between predetermined cognitive structures and experience? To what extent are the structures of spatial cognition universal or how far do they depend on cultural conditions? The argument underlying our reasoning was that it is only by studying the history of spatial thinking that the epistemic status of spatial knowledge can be assessed. We then attempted to substantiate this claim by discussing different aspects of the historical development of spatial knowledge and analyzing the epistemic status of the related structures of spatial thinking. In particular, we encountered the following forms of space:

Naturally conditioned space is structured by elementary mental models controlling action and perception, such as the permanent object model and the landmark model.

Culturally shared space is represented in language, culturally conditioned actions and cultural artifacts, and builds upon the mental structures of naturally conditioned space, endowing them with cultural meaning.

Administratively controlled space is represented by measuring tools, arithmetic and linguistic symbols, and schematic drawings, and adds metric significance to structures of the previous forms of space.

Mathematically reflected space generalizes metric structures by abstraction, using diagrams, formalized language, and other symbol systems for its representation.

Philosophically reflected space generalizes linguistically represented elementary structures by elevating them to the rank of principle and exploring the consequences.

Empirically and disciplinarily imposed space results from the integration of knowledge acquired by systematic observation and experimentation employing conceptual-mathematical formalisms.

A central concern of this chapter was to indicate in which ways these forms of space are genetically related. As we have seen, the occurrence of each new form of space depends on specific socio-cultural conditions. Its concrete realization, by contrast, does not solely depend on these conditions, but also on the cognitive structures it builds upon and on further experience. There is thus always both an aspect of construction and an aspect of experience in these spaces. Both aspects are closely intertwined, of course, because experience is always informed by cognitive structures already present in the mind, and, at the same time, it is experience that shapes the development of cognitive structures. One can thus say that there is no experience that is not structured by the mind, but there is also no mental structure that has not been shaped by experience. Our cognitive structures are the sediments of experience. But sedimentation is a historical process. This is why the understanding of the architecture of cognition requires the historical analysis of its genesis. The different forms of space represent not only successive historical stages, however. They also represent forms of thinking that are simultaneously present within single societies. Different forms of spatial knowledge are shared either by the entire society, or by specialized groups, and may affect each other. Within different societies, they coexist in varied manifestations, each society displaying its unique spectrum of expressions of spatial thinking. The following chapters will highlight this diversity of cultural manifestations of spatial thinking through history and provide ample material for the discussion of their genetic relatedness.

Bibliography

Footnotes