
Fig. 10.1: Taken by Buchwald at Tom and Jehane Kuhn’s home on Memorial Drive in Cambridge in the spring of 1991.
In the fall of 1967 I entered Princeton as a Freshman intending to major in physics but interested as well in history. The catalog listed a course on the history of science, taught by a Professor Thomas Kuhn, with the assistance of Michael Mahoney
During my time as Kuhn’s assistant we would meet every week or two to talk about old physics. He would always emphasize the need to uncover what kinds of characteristic problems were at issue in the past, and about how these problems connected to mathematical and theoretical structures, though not much at the time about experiments proper. In the spring of 1971, Kuhn taught a graduate seminar on the history of thermodynamics. The readings—all of them primary sources—had been carefully prepared and put on reserve. Each week one of the students was responsible for taking the class through the texts. Kuhn did not want a simple summary of relevant issues. He expected you to have figured out precisely what made the text tick. He already had strong notions about the materials, and if you came up with something different from what he had in mind then you had to argue for it line by line, sometimes equation by equation (since most of the texts dealt with in that course were strongly mathematical).
Anyone who encounters Kuhn’s Structure takes away at least the following claims: that scientists working in a given area together hold to a ‘paradigm’ that guides the way they think about their subject, from theory through the design, execution and interpretation of experiments, that group members use the paradigm to solve puzzles as they pursue ‘normal’ scientific work, that problems may eventually fracture the paradigm’s coherence as ‘anomalies’ begin to show, usually from experiments originally undertaken in ‘normal’ research, but perhaps from internal problems affecting consistence, that these may lead some members of the group, or perhaps aspiring entrants, to question basic elements of the scheme, and that, often in a flash of new insight, a ‘revolution’ occurs that replaces the previous paradigm with a new one.
Kuhn’s most detailed effort to work through a body of past physics—his Black-body Theory and the Quantum Discontinuity–appeared in 1978. He had been hard at work on it since 1971. To those who knew him well over the years, the book itself very nicely exemplifies Kuhn's special approach to the history of science as well as his particular views about scientific development. Like most things that he wrote, Black-body Theory generated controversy, some directed at its apparent failure to apply what he had himself laid out in Structure, some directed at his specific, technical claims. It seemed to many of us who knew him that Kuhn was not bothered much or even at all by the former critique, but he was very much concerned with technical criticisms. His need, even compulsion, to find the—not a—core of meaning that unites a disparate series of texts, to extract that largely-implicit structure and to display how it governed and connected to a set of canonical problems, powerfully directed his historical research. Technical criticism accordingly bothered him a great deal, precisely because it went to the core of what Kuhn took to be his central historical task, which was to uncover the hidden integrity of past science.
My own first book (From Maxwell to Microphysics
None of that dealt explicitly with Kuhn’s Structure, but the approach taken was powerfully influenced by his way of treating past science. Much of that was learned directly from him, however, and not pari passu from the Structure itself. Which is perhaps not surprising, since on Kuhn’s account it is only through exemplary situations, often learned directly in the apprentice-like training which students undergo, that one learns how to work a particular system. Neither did From Maxwell claim anything like a ‘revolution’ of the sort that, for example, might be thought to characterize the development of optics at the beginning of the nineteenth century, which was the subject of my second book (The Rise of the Wave Theory of Light, Chicago, 1989).
Perhaps the most important lesson that those of us who studied under Kuhn learned from him, and that does appear, if only implicitly, in the Structure, is that the deepest, most characteristic elements that constitute a field of scientific practice are precisely the ones that are the least obvious and that must be learned through the comparative assimilation of instantiating situations, or what Kuhn came to term ‘exemplars.’ Wave Theory sought explicitly to uncover those unspoken ways of working, and in so doing argued that what appeared on the surface to be the primary points at issue in the debates that eventuated in the theory’s spread were not in fact the principal ones at all.
In the Structure Kuhn had cited the transition to wave optics as an example of crisis producing a revolution, that here we had “Thomas Young’s
Here, then, we have something that is unKuhn-like in one sense—namely in not showing clear signs of anomaly and crisis among the originators of a novel scheme—but very Kuhn-like in another, for these events clearly do indicate that each system had evolved (and quite rapidly so) a striking internal coherence grounded on unstated but firmly held ways of treating problems, ways that showed themselves only through the examination of the exemplary problems that each sought to solve. Reading only prefatory words about the systems, words intended to persuade, almost never reveals the ways in which a system actually works; that can be found only by trying to understand, step by step, how practitioners went about solving problems. This is why Kuhn placed so much emphasis in Structure on back-of-the-chapter problems in physical science and mathematics texts, texts of a sort that first began to appear in the eighteenth century. Trained as a physicist himself, Kuhn was convinced that the only way to learn how to be successful (i.e. to be considered a proper member of the community) was to set up, articulate and solve problems in ways that the community accepted. Training and apprenticeship are consequently often, though hardly always (as, e.g., when a set of practitioners scarcely exists at all), critically important for someone fruitfully to enter an established field.
Kuhn’s move to the Department of Philosophy at MIT in 1979 exemplifies his own sense that the issues with which he was most directly concerned were philosophical in nature, though he remained deeply committed to careful historical understanding, as he conceived it. In 1986 he wrote me a letter that contained the following remark: “I think of my primary talent as a hard-earned ability to read a text, find a way to make it make sense by discovering the conceptual structure that lies behind it. It’s the experience of finding hidden structures that underlies The Structure of Scientific Revolutions and that I’m now back trying to analyze again.” Those of us who studied under him, and many who knew him over the years, will recognize here his distinctive voice and point of view. Voice and view demanded and conveyed an uncompromising, rigorous attempt to push beneath the surface of technical work, to find out how it worked.
Which is why my third book (The Creation of Scientific Effects, Chicago, 1994) explored how Heinrich Hertz
In 1992 I became director of the Dibner Institute for the History of Science and Technology at MIT, where each week a Fellow would give a talk. Tom attended many of these, and once a month or so we would have lunch together. During these last years of his life he was trying hard to develop a lexical understanding of what it is about scientific work that produces difficulties of mutual comprehension between proponents of different systems that ostensibly cover the same phenomenal range. The problem, that is, of incommensurability. Although Kuhn had not lectured in any detail about the idea years ago in my first class with him at Princeton, the core of the notion was certainly there, if not explicitly developed, and those of us taught by him picked up by example what he had in mind.
Many of our talks in the ’90s ranged over examples of that sort of thing, taken not however from such wide-ranging schemes as Ptolemaic versus Copernican astronomy, but from much more limited structures, such as the arguments between proponents of an optics based on waves and those who thought in terms of rays. Or between British developers of electromagnetic fields and their German counterparts. Tom’s evolved understanding orbited about his conviction that the deepest differences between scientific schemes concern the ways in which they respectively divide their universes into kinds of entities. Incommensurability, he thought, was not a vague difference in views, but a specific violation by the one scheme of another’s affiliation among kinds—a violation of the principle that a given kind can be an immediate subset of at most one other. That, it seemed to him, was a general property of scientific systems which captures differences among them. This sounds rather abstract, and it is (partly because Kuhn never developed it into something tied to the roles of instrumentation), but it is nicely descriptive of what seems to be the case historically in a number of cases.
Our discussions in the early ’90s led me at his urging to write a paper explicitly applying the idea to the history of wave optics (Buchwald 1992). We corresponded and talked about the various issues as the paper took shape, and the diagram that it included resulted from our discussions. The dark lines represent the kinds of polarized and unpolarized light that were deployed by those who thought of light in terms of rays in the early 1800s, satisfying the one-immediate-ancestor criterion. The dotted lines show instead how practitioners of wave optics grouped kinds of light together in ways that violated the groupings of ray practitioners. These differences had instrumental consequences that appear quite directly in the literature of the period.
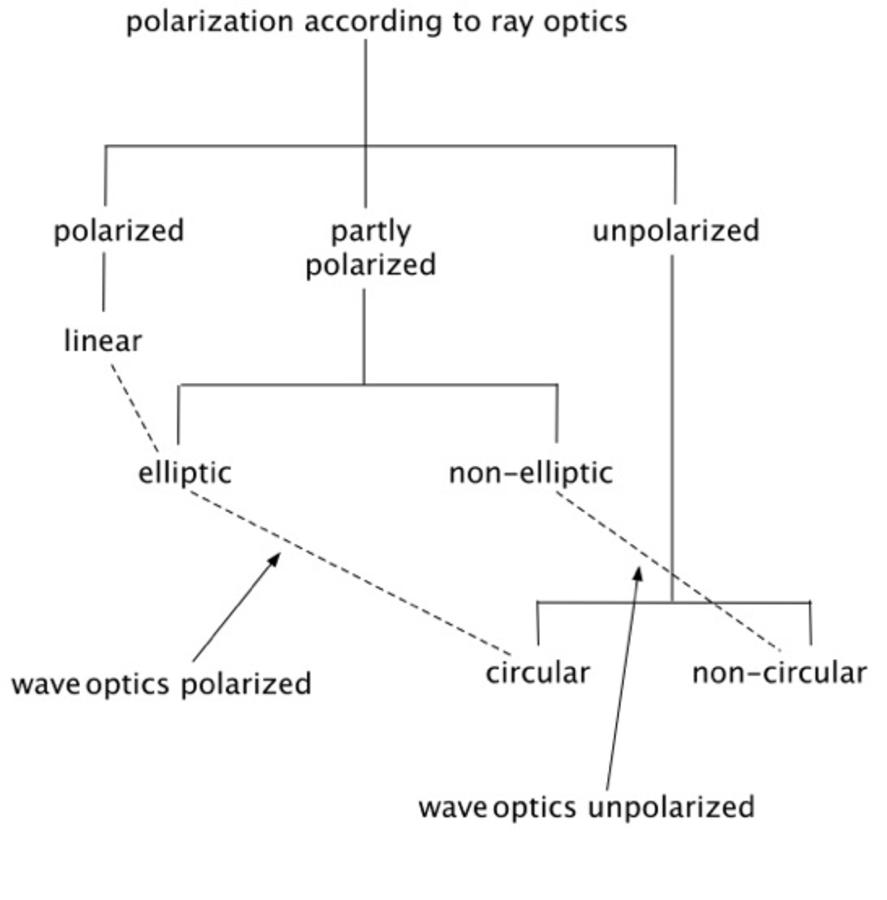
Fig. 10.2: A tree of kinds for light in the early nineteenth century.
Such a system certainly does exhibit the signs of incommensurability, in that a kind term in the one scheme overlapped more than one such term in the other. Similarly, in the histories of electrodynamics that I had studied kind terms involving electric charge and fields or forces crossed disbarred boundaries when trying to apply a term from one scheme to another. And in all of these cases one could find examples in which a practitioner of one scheme, trying to argue against an alternative, or just to use an alternative’s successful results, inevitably worked the alternative scheme in a way that violated the relationships among its entities. That is assuredly an indication of Kuhnian incommensurability, albeit locked down to specifics and avoiding a mushy, global sense of the term that has so often confused or even angered readers of the Structure—though a careful and sympathetic reader can find elements of the notion there as well. In our discussions Tom was interested for the most part in the categorical groupings, less so in their connections to measurement processes, though he did tell me that he intended to think through the latter in more detail in relation to kinds. He never found the time to do so.
Yet it seems to me that instrumentation is critical to understanding the sorting of objects or effects that this way of thinking demands. First, instruments are precisely what divides the elements of the tree from one another: sitting at the nodes or branch-points of the tree, experimental devices assign something to this or to that category. Second, devices may generate new kinds that can either be assimilated by, or that may disrupt, the existing structure. Moreover, experimental apparatus may have its own taxonomic structure that to a very large extent exists apart from that of trees with which it is in other respects associated—provided that experimental relations do not violate otherwise-accepted taxonomies, or at least that incommensurable taxonomies are not brought into contact with one another.
Devices on this account act at the nodes of the tree to assign objects to the appropriate categories. Absent the apparatus there would be no sorting, and the apparatus proper often constitutes an embodiment of the relevant kind-structure. One may very reasonably ask, therefore, whether (in)commensurability, and the doctrine of kinds discussed here, are highly limited in historical application, to, say, science after the late seventeenth century, or perhaps even to science post-1800. What, for example, do kinds have to say about the sort of astronomy practiced by Kepler
This is not an easy question to answer, and I am not certain that the doctrine of kinds can in fact embrace all forms of scientific behavior. It may just be that it is particularly well-adapted to some forms of apparatus-based science. If the doctrine of kinds must be linked to laboratory equipment then their history belongs also to it. I think, however, that a somewhat broader notion of apparatus may extend the utility of the doctrine beyond these boundaries.
‘Apparatus’ naturally suggests—and is so defined by the Oxford English Dictionary—material devices, machines, entities that make things happen to objects or that react to happenings. A signal characteristic of such devices is one’s ability to change them in essential ways, and, in so doing, to make different things happen or to elicit different reactions to the same event. Keplerean astronomy used no such devices, because the telescope cannot work the (celestial) object that is being investigated, nor can it do more than one thing with the object’s (optical) effects. Kepler
From the standpoint of kinds, both forms of apparatus can act as sorters. A slice of crystal in a polarimeter does things to light that assign it to a particular category. One may know almost nothing at all about the crystal’s likely behavior beforehand. Worked properly, the polarimeter produces novel information about the crystal. Theoretical devices can do something similar. Succeeding observations of the loci of a strange heavenly object can be subjected to astronomical theory, and it may as a result become possible to assign it to known categories, e.g. to comets. There is an evident difference between the two cases. The polarimeter acts on the object and sorts it. Astronomical theory acts on something other than the object, something that is itself produced by an instrument that engages an effect of the object. Whereas optical theory does not have to intervene in the polarimeter’s sorting (once the device has been properly built and worked), astronomical theory itself does the sorting work.
Many historical situations exhibit both types. A slice of some transparent stuff may produce colored rings in a polarimeter, thereby assigning it to the class of ring-producing-things. But the rings may not look like ones previously seen, at which point theoretical technology, as it were, comes to bear, yielding in this case a novel class of objects in respect to their optical behavior, namely the class of biaxial crystals. This might even occur without the intervention of much theory through the construction of novel material devices that produce new sortings without violating old connections. If these material and paper attempts at sorting fail, then radical new technologies may be produced, or perhaps the effect may be relegated to the sidelines as something inconsequential. The point is that sorting ‘technologies’ do not have to be physical devices, and this may make it possible fruitfully to use the doctrine of kinds for pre-laboratory science.
The critical role of devices in configuring the taxonomic tree for laboratory science means that taxonomies may be distinguished from one another in two very important ways: first, as to their comparative freedom from device-induced category violations, and second, as to their robustness in respect to novel devices. This is, furthermore, not solely an abstract, philosophical point because scientists often do just that. They are continually using different types of existing apparatus to be certain they have properly understood something, and they generally try to produce new apparatus to get at a process in different ways. A taxonomy that is weak in the first respect and that is not robust in the second will almost certainly not gain adherents over time because it does not work well with or is not fruitful in producing (or both) scientific devices. To the extent that a premium is placed on building a world with apparatus, and on generating new apparatus from that world, such a taxonomy is objectively weak in comparison with one that fits well with existing devices and generates new ones. Nothing in this description requires invoking an absolute, eternal world of entities that apparatus-based science uncovers over time. It does require that, as a matter of fact, devices can be made to work and that new devices can be fabricated as scientific practice grafts, buds and restructures taxonomic trees.
I continue to think that Tom was substantially correct about the importance of incommensurability in scientific practice, and that the concept is best conceived in terms of a tree structure for kinds. Certainly his way of understanding cannot easily encompass the sort of thing that takes place when, say, someone trained as a physicist moves into biology, giving rise perhaps to new regimes with concomitant developments in social, cultural and institutional structures. Though Tom would occasionally talk about such things, he really had very little to say about them in later years since they do not map simply onto issues of incommensurability in the way that he had come to think about the latter. That notion occupied him to the end of his life and, he often told me, constituted his most important contribution to understanding the character of scientific work1.
My fourth book, The Zodiac of Paris (co-authored with Diane Greco Josefowicz, Princeton, 2010) traverses rather different terrain, since here the issues range from archaeological expropriation during the Bourbon Restoration to censorship, religious revanchism, imagined pasts, and the question of who could control antiquity, calculating scientists or philological historians. Still, here too we find Kuhnian traces, since the communities in question usually talked past one another, and even among the computing scientists discord reigned as each group tried to forge its own version of antiquity by means of computations rejected by, and often not understood by, others. In a fifth book, Newton and the Origin of Civilization
Kuhn remains relevant today precisely because he insisted on probing beneath the surface of scientific discourse to reveal the unstated but powerfully operative practices and beliefs that characterize a group. That kind of probing analysis requires immersion in the details of often arcane computations and arguments. Few did it in the past, and few, Kuhn felt, do it today. More’s the pity.
As the years went by Kuhn increasingly found historical research to be difficult. There seem to have been two reasons for his growing reluctance to read or to do history. He had trouble absorbing secondary work, in major part because he brought to histories the same intense commitment to the text’s meaning that he brought to source materials. Vagueness bothered him no end, as did failure to produce the sort of analysis that he found most useful and interesting. But Kuhn was also not himself inclined to grapple with archival materials; he focused almost all of his own historical work on printed works. Yet, and he knew this to be so, the very structures that he so strongly wanted to uncover could often only be excavated from unprinted materials.
References
Buchwald, J. Z. (1992). Kinds and the Wave Theory of Light. Studies in History and Philosophy of Science 23(1): 39-74
Buchwald, J. Z., G. E. Smith (1998). Thomas S. Kuhn, 1922–1996. Philosophy of Science 64: 361-376
- (2002). Incommensurability and the Discontinuity of Evidence. Perspectives on Science 9: 463-498
